Type your answer in the blank and click Check Answer.
Hermione would like to add candy to her little bakery. She decides to sell chocolates and lollipops. She sells chocolates for $0.50 each and she sells lollipops for $1.00 each. She wants this to be only a very small portion of her business, so she would like it to make up no more than $20.00 of her daily sales. Chocolates can go bad more quickly than lollipops, so she limits her production of chocolates to no more than 15 per day. She would like to sell at least 3 lollipops per day, but she won’t make more than 18 in the day.
Hermione makes $0.20 profit for each chocolate sold and $0.65 for each lollipop sold. How many of each candy should she sell to maximize her profits?
Answer:
Hermione should make and sell 4 chocolates and 18 lollipops to maximize her profits.
Let c be the number of chocolates sold and l be the number of lollipops sold. The system for this situation is:

The graph of this system is:
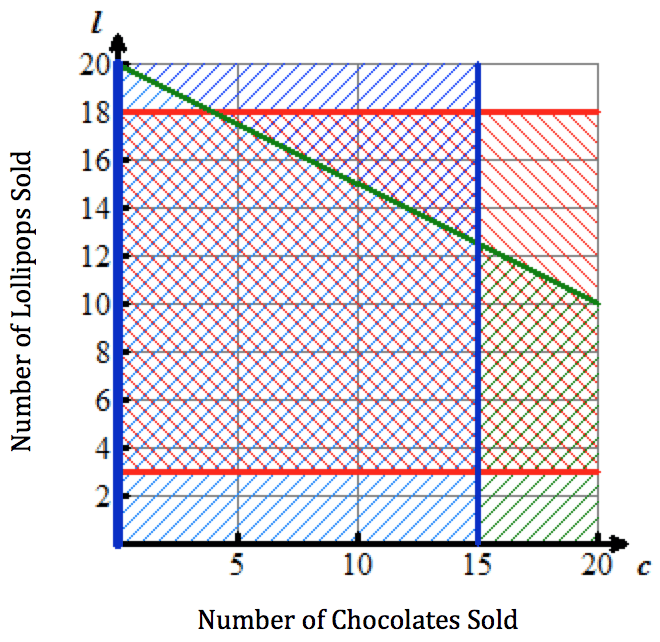
The coordinates of the vertices of the commonly shaded region are:

(0, 3)
(0, 18)
(4, 18)
(15, 12.5)
(15, 3)
Hermione makes $0.20 profit for each chocolate sold and $0.65 for each lollipop sold, so the objective function is P(c, l) = 0.20c + 0.65lP of c comma l equals 20 hundredths c plus 65 hundredths l.
The profit at each vertex is:
P(0, 3) = 0.20(0) + 0.65(3) = 1.95P of 0 comma 3 equals 20 hundredths times 0 plus 65 hundredths times 3 equals one and 95 hundredths.
P(0, 18) = 0.20(0) + 0.65(18) = 11.70P of 0 comma 18 equals 20 hundredths times 0 plus 65 hundredths times 18 equals 11 and 70 hundredths.
P(4, 18) = 0.20(4) + 0.65(18) = 12.50P of 4 comma 18 equals 20 hundredths times 4 plus 65 hundredths times 18 equals 12 and 50 hundredths.
P(15, 12.5) = 0.20(15) + 0.65(12.5) = 11.13P of 15 comma 12 and 5 tenths equals 20 hundredths times 15 plus 65 hundredths times 12 and 5 tenths equals 11 and 13 hundredths.
P(15, 3) = 0.20(15) + 0.65(3) = 4.95P of 15 comma 3 equals 20 hundredths times 15 plus 65 hundredths times 3 equals 4 and 95 hundredths.


