Choose the correct answer(s) and click Submit.
Question 1
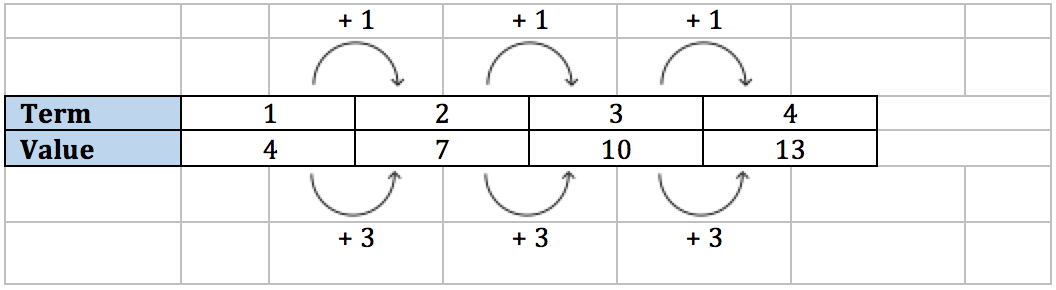
Maria, Leslie, Tammy and Jenn share one secret. Every day, three of the girls each tell one other person the secret. The number of people who know the secret can be described by the pattern shown below.
4, 7, 10, 13, …
Which of the following describes the girls’ secret pattern? Select ALL that apply.
The pattern is an arithmetic sequence with a common difference of +3.
an = a1 + (n - 1)da sub n equals a sub 1 plus the quantity n minus 1 times d.
an = 4 + (n - 1)times⋅3a sub n equals 4 plus the quantity n minus 1 times 3
y = 4 + (x - 1)times⋅3y equals 4 plus the quantity x minus 1 times 3
y = 4 + 3x - 3y equals 4 plus 3x minus 3
y = 3x + 1y equals 3x plus 1
Go to question 2.
Choose the correct answer(s) and click Submit.
Question 2
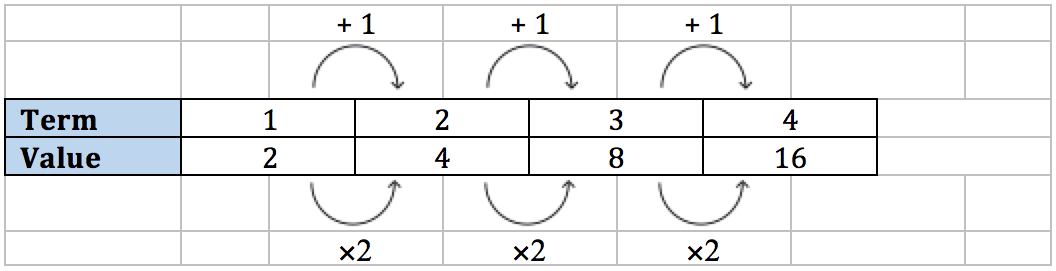
Chris and Jeff also have a secret. On Monday, each of the boys shares the secret with a friend. On Tuesday, the four friends each share the secret with one other person. Every day after that, each person who knows the secret will share it with another person. The number of people who know the secret can be described by the pattern shown below.
2, 4, 8, 16, …
Which of the following describes the boys’ secret pattern? Select ALL that apply.
The pattern is a geometric sequence with a common ratio of 2.
an = a1times⋅rn-1
an = 2times⋅2n-1
y = 2times⋅2x-1
y = 2times⋅2xtimes⋅2-1
y = 2times⋅2xtimes⋅
y = 2x
a sub n equals a sub 1 times r to the power of n minus 1. a sub n equals 2 times 2 to the power of n minus 1. y equals 2 times 2 to the power of x minus 1. y equals 2 times 2 to the power of x times 2 to the power of negative 1. y equals 2 times 2 to the power of x times one half. y equals 2 to the power of x. Go to question 3.
Select an answer from the options below and click Submit.
Question 3
Nathan posts a secret on Facebook, and sixty of his friends read it. However, each day after the post, 
of the friends will forget the secret. The number of people who remember the secret follows a geometric sequence, and can therefore be modeled using an exponential function.
Which of the following geometric formulas and corresponding exponential functions models this situation?
an = 60times⋅
n-1an = 60times⋅(
)n()-1an = 60times⋅(
)n(6)an = 360times⋅(
)n
a sub n equals 60 times one sixth to the power of n minus 1. a sub n equals 60 times one sixth to the power of n times one sixth to the power of negative 1. a sub n equals 60 times one sixth to the power of n times 6. a sub n equals 360 times one sixth to the power of n.↓ ↓
y = 360times⋅(
)x
y equals 360 times one sixth to the power of x
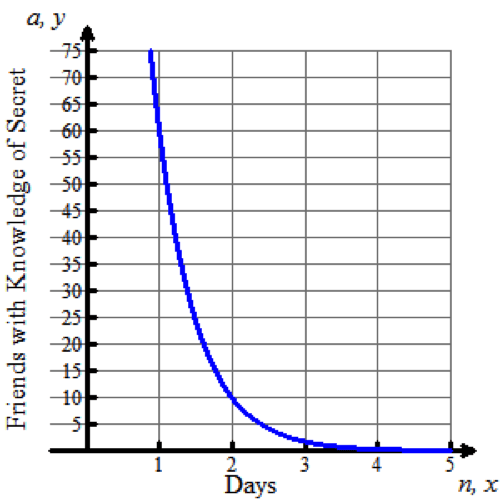
(This is exponential decay because the absolute value of the common ratio is less than one.)
Go to question 4.
Select an answer from the options below and click Submit.
Question 4
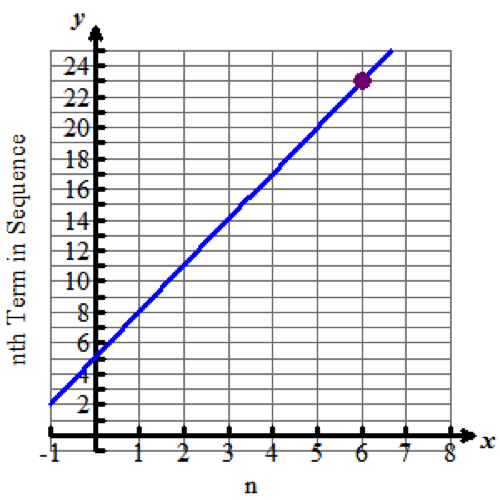
The two arithmetic representations model the growth of a rumor spread around a school. Which sequence has the greater 6th term?
The sixth term for an=53+(n-1)(-negative7)a sub n equals 53 plus the quantity of n minus 1 times negative 7 would be a6=53+(6-1)(-negative7)=18a sub 6 equals 53 plus the quantity of 6 minus 1 times negative 7 equals 18.
Extending the graph for answer choice B shows the sixth term to be 23.
